How Vibration Monitoring Works

Vibration monitoring is a tried and tested method of monitoring the condition of critical machinery and plant, so that preventative measures can be taken to avoid costly breakdowns and unnecessary maintenance runs.  

What is Vibration? 

A body is said to vibrate when it describes an oscillating motion about a reference position. 

Velocity and acceleration are the 2 attributes that are most closely associated with vibration. 

If we assume that the oscillating motion of vibration is sinusoidal, then there are only 3 questions you need answers to in order to select an accelerometer: 

1. What is the approximate amplitude of the vibration in millimetres? 
2. What RPM or frequency does the machine running at during normal operation? 
3. Do you want to monitor velocity (mm/s), or acceleration (mm/s2 or g)? 

The profile of vibration is more complex than a pure sinusoidal waveform, but this is a good place to start, when calculating what accelerometer is required.  It is also worth noting that when a smooth-running machine starts succumbing to wear and tear, the numbers are going to get bigger in terms of amplitude and frequency. 

What follows is a brief explanation how we arrive at the following simple equations to work out the velocity in mm/s and acceleration in or g of pure sinusoidal waveforms. 

If we can get the amplitude A and frequency f from the customer, then this gives us an idea of the starting point of the measurement range we should be looking at when it comes to recommending the right accelerometer for the application. 

Equations to Calculate Velocity and Acceleration regarding Vibration 

You can use the following simple equations to work out the velocity in mm/s and acceleration in or mm/s2 g of pure sinusoidal waveforms. 

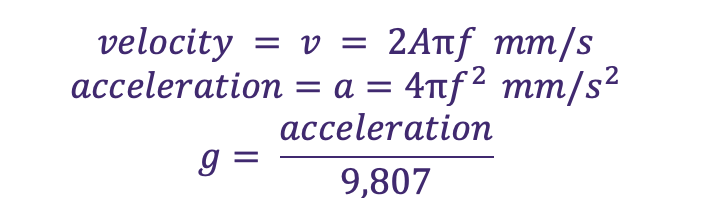
The Mathematics of Vibration Monitoring

Let’s assume the vibration is sinusoidal in nature. 

A sine wave is described mathematically by the equation: 

Simplifying the Mathematics of Vibration 

At this stage, we are not interested in the displacement, velocity and acceleration at a particular instant in time.  You just need to know the maximum values of each of these. 

The maximum value a sine and cosine function is 1.  So, to find the maximum value of the displacement, velocity and acceleration in the above equations we let sin(2 pi f×t)=1 and cos(2 pi f×t)=1, so we get the following result: 

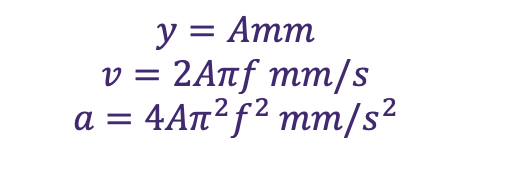
For example, let’s plug in some values and assume your machinery is running at 1,200 RPM (frequency = 20 Hz) and the peak to peak vibration is 2 mm, therefore A=1.

Our answers to the above equations are: 

For acceleration, the specifications for this parameter are always given in g…  To find g, we need to divide the acceleration by gravity, which is 9,807 mm/s2.  Therefore, 

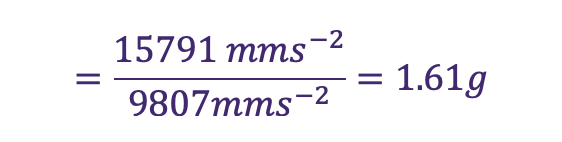
So, to repeat, if you can answer the two questions: 

1. What is the approximate amplitude of the vibration in millimetres? 
2. What RPM does the machinery normally run at? 

then you can calculate the velocity and acceleration of the application, which then helps you choose an appropriate accelerometer. 

Remember, the above results are the maximum values for the amplitude and frequency of a sinusoidal signal.  The actual output from the signal conditioner will probably be RMS value, which is: 

ADM has published a calculator, which will give velocity, acceleration and g. This can be downloaded by clicking on the following link: 

Download Calculator for Velocity and Acceleration

How are Velocity and Acceleration Physically Measured? 

To understand the technologies required to measure velocity and acceleration in vibration let’s revisit the two equations again: 

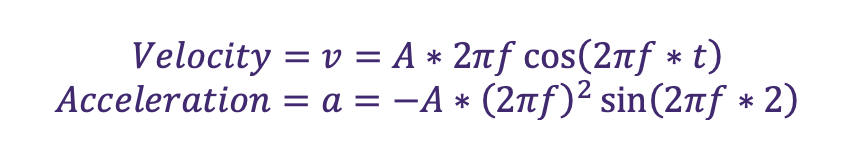
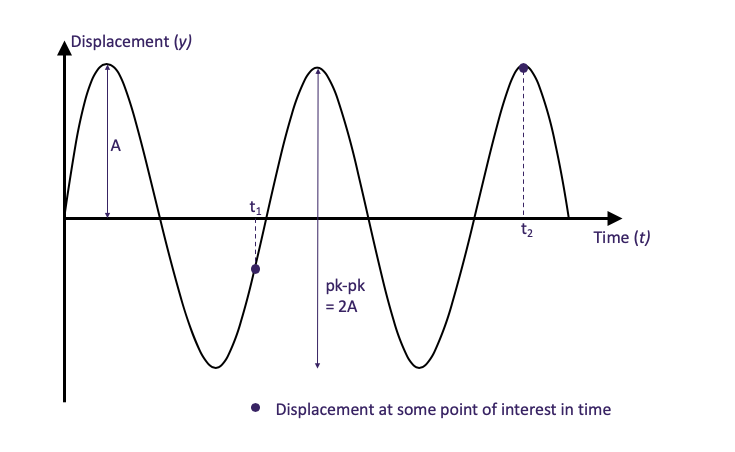
Let’s say our vibration has an amplitude A of 1 mm and the frequency f is 2 Hz as shown in the graph below: 

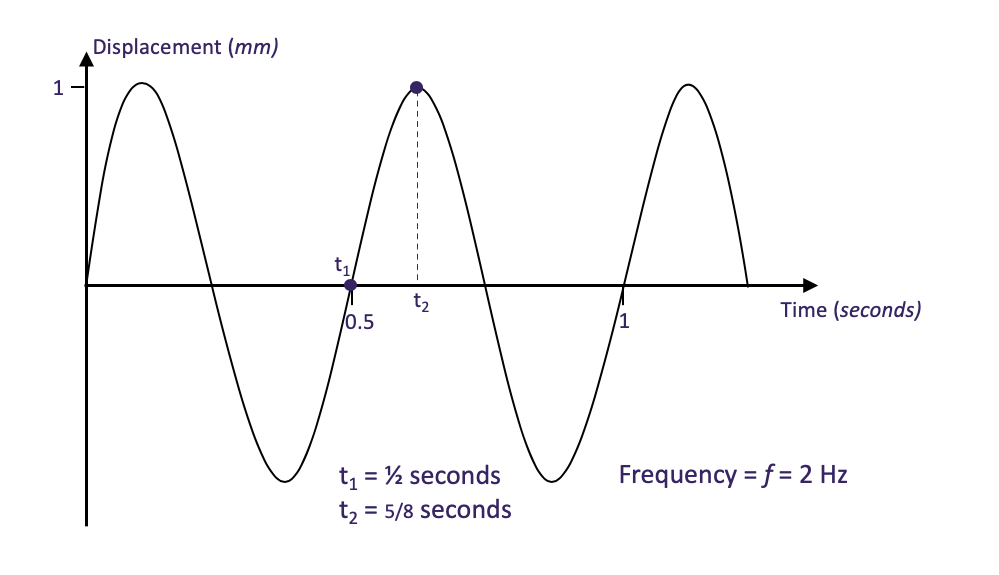
We want to know the velocity and acceleration of the body after ½ second ( ).  Substituting these values into the equations gives: 

Now let’s have a look at the velocity and acceleration at seconds:

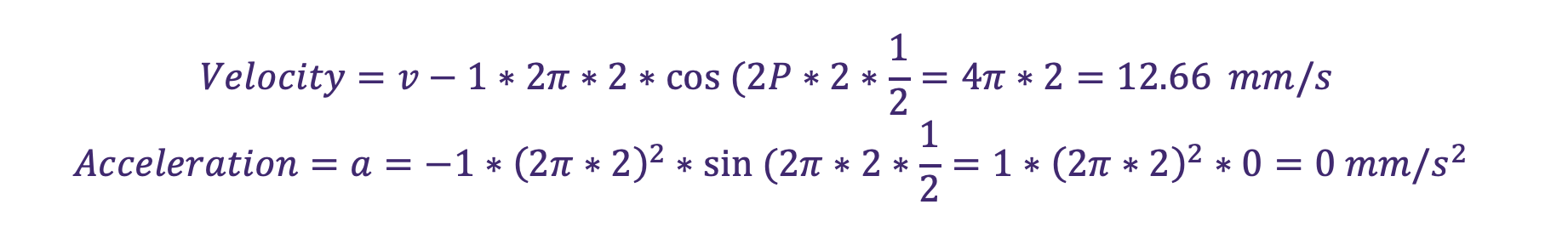
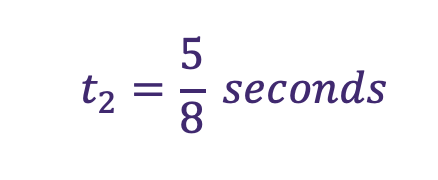
We can see from this example that when the velocity is maximum, the acceleration is zero, and when the acceleration is maximum, the velocity is zero. 

So, you can’t really use the same technology to give both velocity and acceleration, their magnitudes are very different, and they are 90ᴼ out of phase with each other.  Each must have its own technology, but because they are interrelated, there are ways of using just one technology to derive both velocity and acceleration.  It is shown how a little later. 

How Do Accelerometers Work?

Velocity 

A common way to measure velocity is to use a magnet suspended within a coil of wire.  When the magnet is moving, a voltage is generated across the ends of the coil.  The faster the coil moves, the higher the voltage.  

The velocity of vibration is maximum when the oscillation passes through the zero reference, and this is when the voltage output from the magnet and coil is maximum. 

The instrument that purely measures velocity is called a velocimeter, and not an accelerometer. 

Acceleration 

A common way to measure acceleration is to use a piezo-electric crystal under pressure from a weight.  When the sensor receives a shock force (i.e. apply an impulse), the weight stays still whilst the sensor accelerates causing the crystal to deform.  We know that when you deform a crystal, a charge is generated across its opposite faces, which is then amplified by a charge amplifier to give an output voltage.  The picture below gives a reasonable illustration of the principle, where the inertial mass is “free” to move and apply a force on the crystal, therefore causing it to deform. 

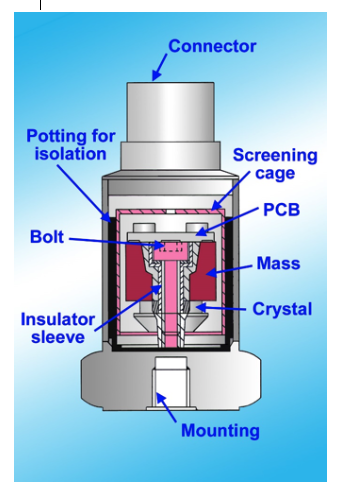
The maximum charge generated by the crystal is when it has been deformed the most, which occurs when the acceleration is maximum, i.e. at the peaks and troughs of the oscillation. 

This instrument is correctly called an accelerometer. 

So why is Velocity measured by an Accelerometer?

Well, it’s back to the calculus.  We saw that you can determine Acceleration by differentiating the equation for Velocity. 

To get back to Velocity when you have Acceleration, you need to integrate the acceleration equation: 

For the sinusoidal vibration that we have been analysing, the above integral returns: 

as before. 

In electronics, a capacitor across the input of a signal amplifier circuit is used to give us an integrated output.  In software an integration function is applied to the acceleration to derive the velocity.  So, when we see that an accelerometer is being used to give a velocity measurement, we know that some transformation of acceleration to velocity is being done in the sensor, or the signal conditioner. 

The opposite can also apply when we see a velocity sensor giving us an acceleration output derived from a calculation.  To do this, a capacitor is used in series with the input signal, which serves as an electronic differentiator, or a derivative function is applied in software to achieve the same outcome. 

ADM Instrument Engineering offers the Monitran range of accelerometers, keeping a select range in stock. ADM has access to the entire Monitran range, so if you can't find what you are looking for please call our office on 1300 236 467.

Multiple Output Signal Conditioning 

The Monitran MTN/8065 Tri-Output Vibration Interface Module takes the raw AC output signal from an accelerometer and gives 3 concurrent measurements: 

• RMS Velocity (0 – 10 volt output) 
• Acceleration (mV/g) 
• Enveloped g Output (0 – 10 volt output) 

The first two have already been discussed, so what is Enveloped G? 

The signal conditioner takes the acceleration output and the applies Fast Fourier Transformation to it to generate the enveloped G output.  What is FFT? 

Take a step back.  Mr Fourier discovered that every signal in the world that is periodic (i.e. has repetition) can be built up from sinusoidal signals of various amplitudes and frequencies all mushed together.  Voila, the electronic synthesiser was born.  His transformation is also used to take what appears to be a very noisy signal, like vibration, separate out the noise, and identify the underlying signals that are not noise in terms of their fundamental and harmonic frequencies. 

Enveloped g is now widely used in vibration monitoring to separate out the fundamental frequency and a few of the harmonics using filtering and superimposing them into one signal. 

If, for instance, a bearing on the machine is failing, it has a small fracture in the race, and every ball rolling over the fracture vibrates.  The vibration will have a fundamental frequency as well as harmonics.   The FFT picks these out and you will be able to see, much earlier than with a normal acceleration signal, that something in the machine needs attention as the enveloped g signal will appear above the background noise much earlier than without applying the technique. 

Further Information on Vibration Monitoring and Vibration Sensors

If you would like to know more about vibration measurement and using accelerometers to monitor the condition of your plant and machinery, then please do not hesitate to contact ADM Instrument Engineering. 

A member of our expert team will gladly answer any questions that you may have.

IS THIS INFORMATION USEFUL?

If so, why not share it with your peers and colleagues. Simply click on the blue LinkedIn share icon below.